Several articles of H.Nakamura

Some recent papers:

H.Nakamura, R.S.Tarmidi
"On a two-parameter family of tropical Edwards curves"
Kyushu J. Math. 78 (2024), 373--393.

H.Nakamura, D.Shiraishi
"Landen's trilogarithm functional equation and l-adic Galois multiple polylogarithms"
Preprint 2022

H.Nakamura
"Demi-shuffle duals of Magnus polynomials in a free associative algebra"
Algebraic Combinatorics Volume 6 (2023) no. 4, pp. 929-939.

DOI: 10.5802/alco.287
PDF [December 2022]
arXiv:2109.14070 [math.NT] (Preprint [29/09/2021 --])

A.Minamide, H.Nakamura
"The automorphism groups of the profinite braid groups"
American Journal of Mathematics,
144(5) (2022) 1159--1176.

H.Nakamura:
"Arithmetic and Combinatorics in Galois fundamental groups"
(in Proceedings of the RIMS workshop
``Profinite monodromy, Galois representations, and Complex functions''
held at Kyoto University on May 21--23, 2018)
RIMS Kokyuroku 2120 (2019), 188--198.

pdf (130kb)
---
( related Japanese article PDF)

H.Nakamura, Z.Wojtkowiak
"On adelic Hurwitz zeta measures"
Journal of the London Mathematical Society 101(3),(2020),938-959.
DOI: 10.1112/jlms.12292

H.Nakamura
"Moving frames and Eisenstein invariants"
in "Various Aspects of Multiple Zeta Values 2016" (H.Furusho ed.)
RIMS Kokyuroku, Vol.2015, (2017), pp.162-169.

H.Nakamura, Z.Wojtkowiak
"On distribution formula for complex and l-adic polylogarithms"
in "Periods in Quantum Field Theory and Arithmetic"
(J.Burgos Gil, K.Ebrahimi-Fard, H.Gangl eds),
[Conference proceedings ICMAT-MZV 2014]
Springer Proceedings in Mathematics & Statistics 314 (2020), pp.593--619.

H.Nakamura
"On profinite Eisenstein periods in the monodromy of universal elliptic curves"
Preprint, January 2016:

H.Nakamura, K.Sakugawa, Z.Wojtkowiak
"Polylogarithmic analogue of the Coleman-Ihara formula, II"
RIMS Kokyuroku Bessatsu B64 (2017), 33-54.

H.Nakamura, K.Sakugawa, Z.Wojtkowiak
"Polylogarithmic analogue of the Coleman-Ihara formula, I"
Osaka J. Math. 54 (2017), 55--74.

Link to Project Euclid Open Access

H.Nakamura:
"On mono-nodal trees and genus one dessins of Pakovich-Zapponi type",
Tokyo J. Math. 39 (2017), 783--795.

Link to Project Euclid (Full-text: Open Access)

Articles on Anabelian Geometry

H.Nakamura, A.Tamagawa, S.Mochizuki:
``The Grothendieck Conjecture on the Fundamental Groups of Algebraic Curves''
Copyright 1999 American Mathematical Society
``Sugaku Expositions'' (AMS), Volume 14 (2001), 31--53
English translation (by S.Mochizuki) from ``Sugaku'' 50(2), 1998, pp. 113-129 (Japanese).

Y.Ihara, H.Nakamura:
``Some illustrative examples for anabelian geometry in high dimensions''
in `Geometric Galois Actions I' (L.Schneps, P.Lochak eds.)
London Math. Soc. Lect. Note Series 242 (1997), pp. 127--138.

H.Nakamura:
``Galois representations in the profinite Teichmueller modular groups''
in `Geometric Galois Actions I' (L.Schneps, P.Lochak eds.)
London Math. Soc. Lect. Note Series 242 (1997), pp. 159--173.

H.Nakamura :
"Galois rigidity of profinite fundamental groups"
Copyright 1997 American Mathematical Society
Sugaku Expositions (AMS) 10 (1997), no. 2, 195--215.
English translation from Sugaku 47 (1995), no. 1, 1--17.

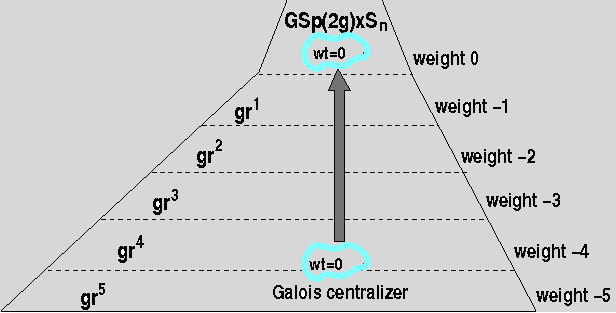H.Nakamura:
"On Galois rigidity of fundamental groups of algebraic curves"
in "Nonabelian Fundamental Groups and Iwasawa Theory"
(J.Coates, M.Kim, F.Pop, M.Saidi, P.Schneider eds.)
London Math. Soc. Lecture Note Series, 393 (2012), 56--71 (Cambridge UP).

pdf
This is a translation into English of an old Japanese article published in
"Report Collection of the 35th Algebra Symposium held at Hokkaido University in 1989"
+ 8 complementary notes newly added in English.

Galois-Teichmueller theory:

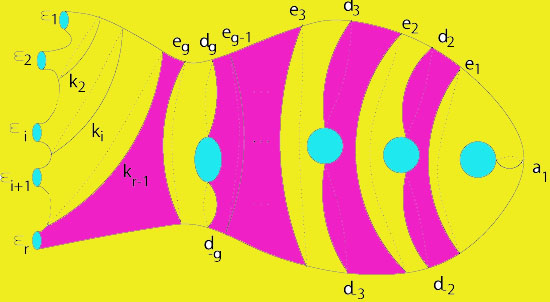
H.Nakamura :
``Limits of Galois representations in fundamental groups along maximal degeneration of marked curves II''
Proc. Symp. Pure Math., 70 (2002), 43--78

ps / pdf

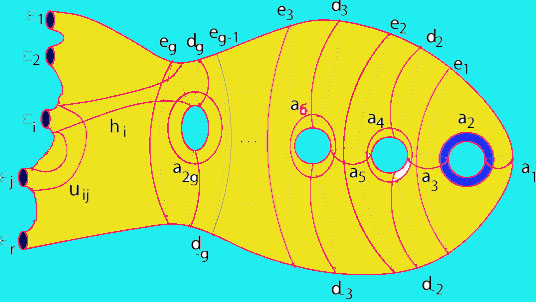P.Lochak, H.Nakamura, L.Schneps:
"Eigenloci of 5 point configurations on the Riemann sphere and the Grothendieck-Teichmueller group"
Math. J. Okayama Univ. 46 (2004), 39--75.

H.Nakamura, H.Tsunogai:
"Harmonic and equianharmonic equations in the Grothendieck-Teichmueller group, II"
in "Primes and Knots" (T.Kohno, M.Morishita eds.)
AMS Contemporary Mathematics 416 (2006), 197--211

dvi / pdf

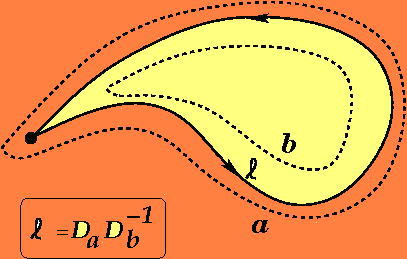H.Nakamura, H.Tsunogai, S.Yasuda:
"Harmonic and equianharmonic equations in the Grothendieck-Teichmueller group, III"
Journal Inst. Math. Jussieu 9 (2010), 431-448.

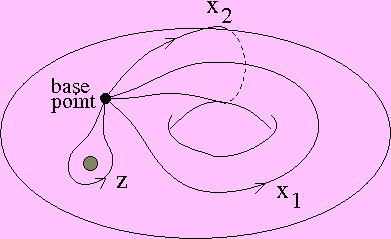NTY2010jimj.pdf (Copyright: Cambridge University Press)
available from Cambridge Journals Online

Arithmetic functions:

H.Nakamura:
``Tangential base points and Eisenstein power series''
in ``Aspects of Galois Theory'' (H.Voelklein, D.Harbater, P.Mueller, J.G.Thompson, eds.)
London Math. Soc. Lect. Note Series 256 (1999), 202--217.
In the 1st printing, figures on p.204, p.213 had been unfortunately dropped after editorial process.
A corrected version is available here:

H.Nakamura:
``Generalized Rademacher functions and some congruence properties''
in ``Galois theory and modular forms'' (K.Hashimoto, K.Miyake, H.Nakamura eds.)
Developments in Math. Vol.11 (2003), pp.375--394, Kluwer Acad. Publ.

dvi / pdf

H.Nakamura, Z.Wojtkowiak:
"Tensor and homotopy criteria for functional equations of l-adic and classical iterated integrals"
in "Nonabelian Fundamental Groups and Iwasawa Theory"
(J.Coates, M.Kim, F.Pop, M.Saidi, P.Schneider eds.)
London Math. Soc. Lecture Note Series, 393 (2012), 258--310 (Cambridge UP).

H.Nakamura:
"On arithmetic monodromy representations of Eisenstein type in fundamental groups of once punctured elliptic curves"
( Publ. RIMS, Kyoto University 49 (2013), 413--496.

pdf (84p.)
An earlier compiled version appeared in RIMS Preprint Series as RIMS-1691, February 2010.

H.Nakamura:
"Some congruence properties of Eisenstein invariants associated to elliptic curves"
in "Galois-Teichmueller theory and arithmetic geometry"
-- Proceedings of conferences in Kyoto 2010
(H.Nakamura, F.Pop, L.Schneps, A.Tamagawa eds.)
Advanced Studies in Pure Math. 63 (2012), 813--832.

Elementary Moduli Space:

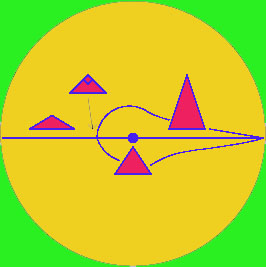
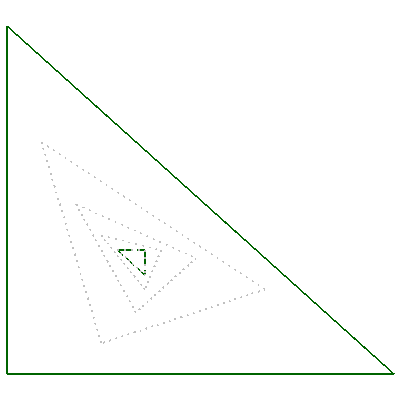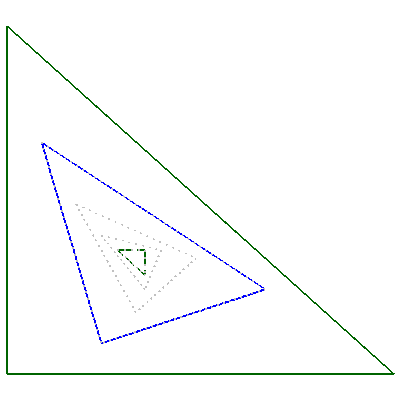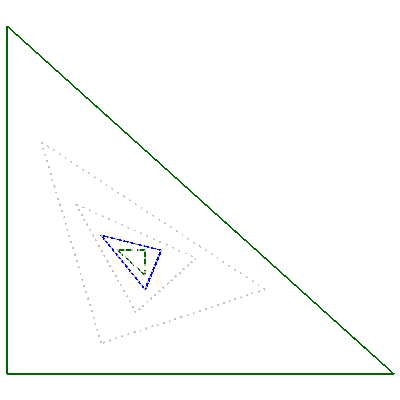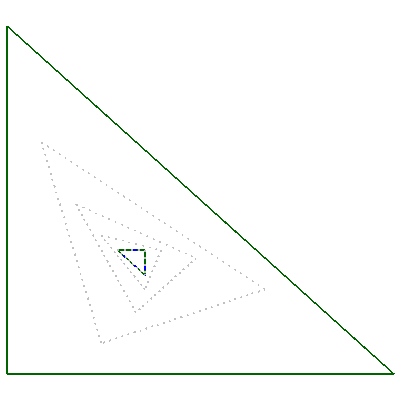H.Nakamura, K.Oguiso:
``Elementary moduli space of triangles and iterative processes''
--- a revised version of a preprint written in 1987
J. Math. Sci., Univ. Tokyo, 10 (2003), pp. 209--224

N.Kanesaka, H.Nakamura:
"On hyperbolic area of the moduli of $\theta$-acute triangles"
Math. J. Okayama Univ. 55 (2013), 191--200.

H.Nakamura, H.Ogawa
"A family of geometric operators on triangles with two complex variables"
Journal of Geometry 111, Article number: 2 (2020)
DOI: 10.1007/s00022-019-0514-y

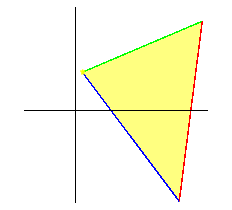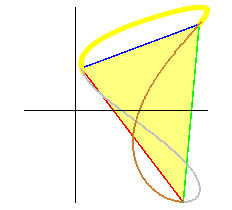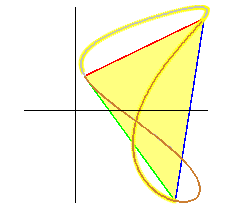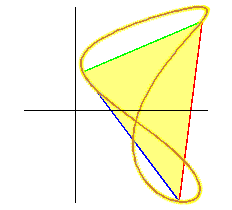
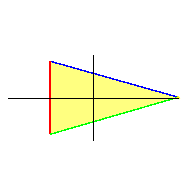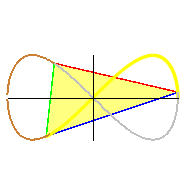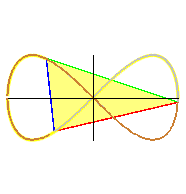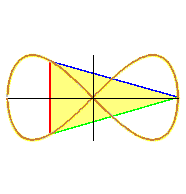
H.Nakamura, H.Ogawa
"On generalized median triangles and tracing orbits"
Results in Math. 75, 144 (2020).

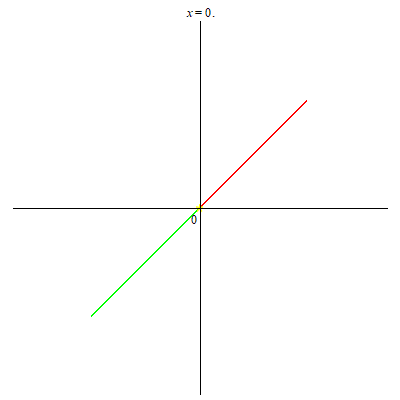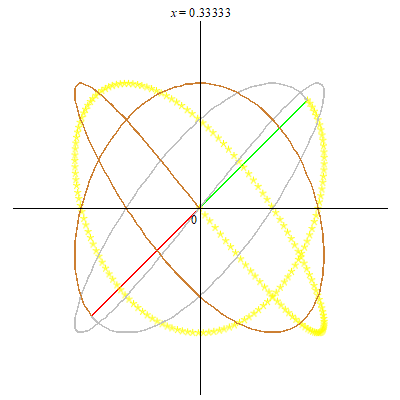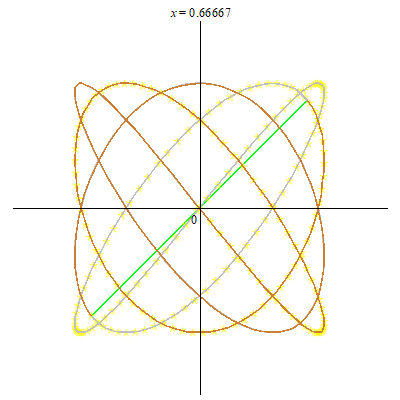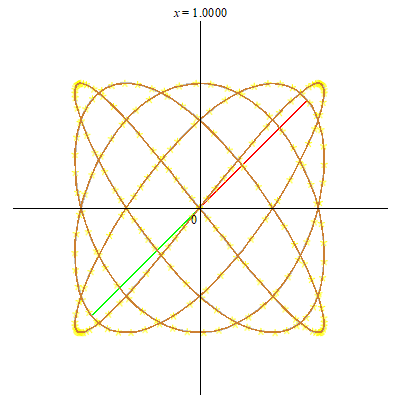
E.Kin, H.Nakamura, H.Ogawa
"Lissajous 3-braids"
Journal of Math. Soc. Japan 75(1)(2023), 195--228.

More could be available via Email: nakamura((at))math.sci.osaka-u.ac.jp

Go back to Front Page